CHAPTER 9 : WAVE MOTION

The transfer of energy in an elastic medium, without the net transport of the medium, occurs through the propagation of waves. The waves are formed due to the oscillation of the particles in the medium which, in succession, transfer the energy from one particle to another due to the elastic forces holding them together.

9.1 Transverse waves and Longitudinal waves

Transverse Waves

When the wave propagates along the transverse (perpendicular) direction of the oscillation of the particles, then it is called transverse Wave.

The diagrams represent schematically the formation of a Transverse Wave. 0,1,2,3,4 particles of an elastic medium that are equidistant from each other and capable of vibrating along the vertical with the same amplitude (r), period (T). At t = 0, the particle begins to vibrate, indicated by 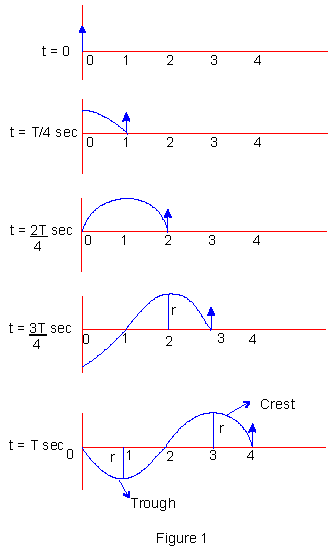. After T/4, 2T/4 , 3T/4, T the arrow shifts to 1^st to 2^nd to 3^rd to 4^th particle, due to the transfer of energy from one particle to another .

The characteristics of transverse waves are :-

1. Formation of troughs and crests.

2. Successive crests or troughs are separated by constant distance called wave length.

3. The wave progresses with velocity where T is the period of wave in interval of time in which the wave advances by

4. Frequency ( n) of waves is defined as number of waves crossing any point in the medium per unit time i.e.

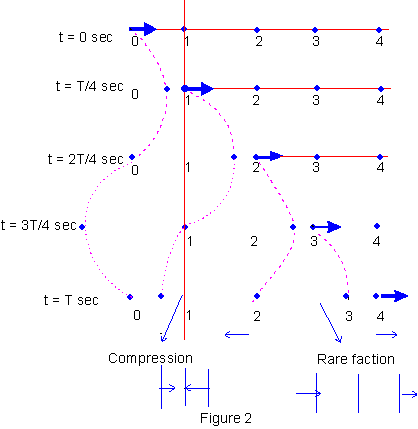
Longitudinal Waves

The dashed curves represent the position of the particles at different moments.

Click here to enlarge

When the propagation of waves occurs along the same direction as that of the oscillations of the particles, then a longitudinal wave is formed. The diagrams above represent the formation of longitudinal waves.

The characteristics of these waves are: -

1. Formation of compression and rarefaction .

2. The distance between successive compressions or rare factions is called wave length l .

3. The wave propagates with velocity T is period of wave.

4. Frequency of waves is n =
   \ v = n l

[next page]

Index

9.1 Tranverse and Longitudinal Waves
9.2 Equation of Linear, Progressive, Harmonic Waves

Chapter 10