Example 20 Find the volume of a sphere of radius ' r'
Solution : Consider the sphere as generated by revolving the upper half of the circle x2 + y2 = r2 about the x-axis (see figure). Clearly x-axis is the boundary of this planar region. We can use disk method here
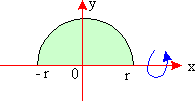
The volume of the sphere is
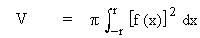
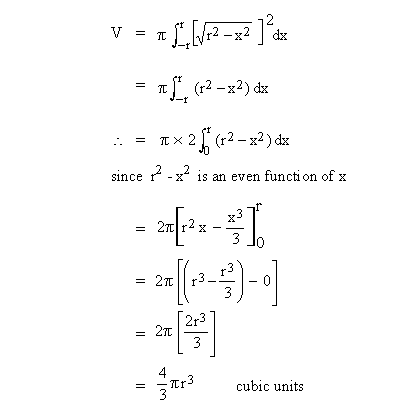
Example 21 Find the volume generated revolving the curve y2 = x2 - x4 about the x-axis.

Solution : From the adjoining figure, it is clear that the required volume is twice that is generated by revolving the area of the region bounded by the curve in the lst quadrant about the x-axis. The boundary of this curve is clearly the x-axis. Therefore using disk method, we have
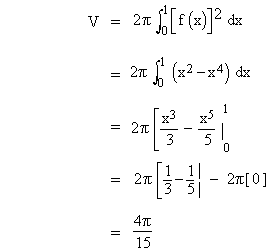
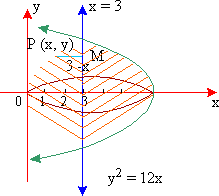
Example 22 Find the volume generated by revolving the area bounded by the parabola y2 = 12x and its latus rectum about the latus rectum.
Solution : Now latus rectum of the parabola y2 = 12x which is the line x = 3 is the axis about which the planar area (see figure) is revolved to generate the required volume, clearly by disk method, we use
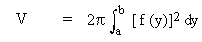
Here the disk is of radius (3 - x) units and the limits of integration are obtained by putting x = 0 in y2 = 12x Þ
y = 0 and x = 3 Þ
y = 6 i.e. the altitude or thickness of the disk is 6 units.
\ The required volume (of revolution) is
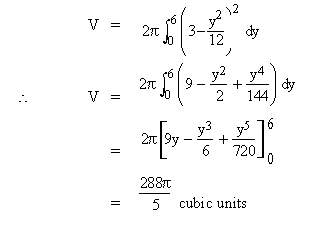 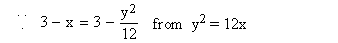 
Washer Method :
We use this method in the situation where the axis of revolution is not the boundary of the planar region. To find the volume of the solid, cross sections are taken perpendicular to the axis of revolution.
A washer is a circular disk with a hole in it. In other woods a small disk is removed from the larger disk, noting that they are concentric disks of equal thickness.
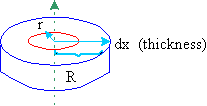
Let 'R' be the radius of the larger disk and 'r' be the radius of the smaller one (see figure). Then the circular face area of this washer is p
(R2 - r2)
Thus its volume will be p
(R2 - r2) dx. Here we can express R and r as the functions of x or y depending upon the axis x or axis y, to whichever it is perpendicular. Therefore if the area is bounded by y = f(x) and y = g(x) with f (x) ³
g (x) on [a, b], then the volume (V) of revolution of this planar region about the x-axis is given by
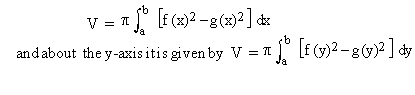
[next page]
|