4.5 Mode
It is the size of that item which possesses the maximum frequency. According to Professor Kenney and Keeping, the value of the variable which occurs most frequently in a distribution is called the mode.
It is the most common value. It is the point of maximum density.
Ungrouped Data
Individual series : The mode of this series can be obtained
by mere inspection. The number which occurs most often is the mode.
Example Locate mode in the data 7, 12, 8, 5, 9, 6, 10, 9, 4, 9, 9
Solution : On inspection, it is observed
that the number 9 has maximum frequency. Therefore 9 is
the mode.
Note that if in any series, two or more numbers have the maximum frequency, then the mode will be difficult to calculate. Such series are called as Bi-modal, Tri-modal or Multi-modal series.
Grouped Data
Steps :
-
Determine the modal class which as the maximum frequency.
By interpolation the value of the mode can be calculated as -
Mode = 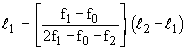
where

Example
Calculate the modal wages.
Daily wages in $ : 20 -25 25-30 30-35 35-40 40-45 45-50
No. of workers : 1
3 8
12 7
5
Verify it graphically.
Solution:
Here the maximum frequency is 12, corresponding to the class interval (35 - 40) which is the modal class.
Therefore 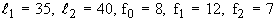
By interpolation
Mode = 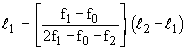
 Modal wages is $37.22
Modal wages is $37.22
|